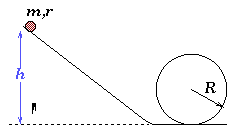
Solution: We have solved this problem in the past by assuming that
the object slid without friction along the track. The condition for
maintaining contact with the track is unchanged from that problem. At every
point along the path, the forces acting on the ball (the gravitational force,
the normal force of the track, and the frictional force) must act so as to
satisfy the centripetal condition. Since the velocity of the center-of-mass
will be lowest at the top of the loop and the normal and gravitational forces
will both be pointing down there, this point sets the condition for
maintaining contact with the track (see the figure below).

To maintain a circular path along the track, at the top of the loop we must
have
The minimum height, h, corresponds to the minimum velocity at the top of the loop since we know it is the work done by gravity that imparts kinetic energy to the ball. In turn, the minimum velocity at the top of the loop corresponds to FN = 0. So, mvtop, min2 = mgR ==> vtop, min2 = gR.
For rolling without slipping, mechanical energy is conserved (since static
friction on the ball does no work), so, at the top of the loop
| Wgrav | = Ktrans. + Krot. |
| mg(h - 2R) | = ½ mvtop2 + ½ I*omegatop2 |
For the minimum height, h, we need to set vtop to vtop,
min and to note that v = omega*r for rolling without slipping. Thus,
| mg(h - 2R) | = ½ mvtop, min2 + ½ I*omegatop2 |
| = ½ mvtop, min2 + ½ (2/5 mr2)(vtop, min/r)2 | |
| mg(h - 2R) | = 7/10 mvtop, min2 |
| g(h - 2R) | = 7/10 gR ==> |
| h | = 7/10 R + 2R = 2.7R |
We note that this result for the minimum h is higher than in the case of a sliding object (2.5 R) since some of the work done by gravity goes into rotating the ball, and the center-of-mass therefore goes more slowly for rolling than for sliding.
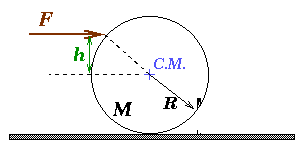
Solution: First, we need to make some reasonable assumptions to
simplify the problem. We assume that the force of the cue stick on the ball is
large and over a very short period of time so that, for this time period, the
change in the translational motion of the ball is determined exclusively by
the cue stick force. In other words, we will ignore the frictional force
acting on the ball during the time the cue stick is acting. Second, since
rolling without slipping is to begin immediately, we assume the instantaneous
acceleration of the center-of-mass, a, is equal to alpha*R. If we consider the
torque about an axis through the center-of-mass and perpendicular to the page,
then, assuming that the frictional force is negligible for the short time that
the cue stick force is acting, we can get the torque acting about this axis
from the diagram below
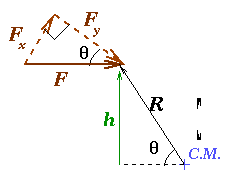
Only the component of the force which is perpendicular to the line
connecting the point where the force acts and the axis of rotation contributes
to the angular acceleration. This component is shown as Fx. The torque is
| Fsin(theta)*R | = I*alpha |
| F*R*sin(theta) | = (2/5)MR2*a/R |
| Fh | = (2/5)MRa |
The acceleration of the center-of-mass during the time F is acting is a = F/M if we
assume the frictional force is negligible during this time. So, using this in
our previous result
| Fh | = = (2/5)MRa |
| = (2/5)MR*(F/M) ==> | |
| h | = 2/5 R |
So we want to hit the cue ball at a point 2/5 R above the center. Hitting below this point causes the ball to slide some distance before rolling without slipping. Hitting above this point causes the cue ball to have top spin. It spins faster than it should and has to slow its rotation rate before rolling without slipping can begin.
Solution: First, we note from our previous problem that if we hit
the ball at a height less than 2/5 R above the center, then rolling does not
begin immediately. In fact, if we hit the ball dead center, then the ball
begins sliding with no initial angular velocity, hence omega0 = 0.
As the ball slides, the only force acting in the horizontal direction on it is
the friction. This frictional force causes a torque fR
= I*alpha. We can not assume that alpha = a/R while the ball is
sliding, however, the condition for rolling without slipping to begin is the
time at which v = omega*R. Thus the initial velocity and angular velocity are
v0 and 0, respectively, and the final velocity and angular velocity
are v and omega where v = omega*R.
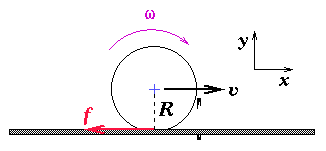
The angular velocity as a function of time can be found once the angular
acceleration is known, so
| fR | = I*alpha |
| muMgR | = (2/5)MR2alpha ==> |
| alpha | = (5/2)mug/R |
Let's say that rolling without slipping begins at a time t. The angular
velocity after a time t is
But, by definition of t, omega = v/R, so
Now, the translation equation of motion gives us the deceleration of the
center-of-mass velocity
Since this acceleration is constant, we can use one of the equations for
motion to relate the initial and final velocities
| v | = v0 + at |
| (5/2)mugt | = v0 - mugt ==> |
| (7/2)mugt | = v0 ==> |
| t | = 2v0/(7mug) |
The distance traveled in this time is
| d | = v0t - ½ at2 |
| = v0[2v0/(7mug)] - ½ (mug/2) [2v0/(7mug)]2 ==> | |
| = 2v02/(7mug) - (2/49) [v02/(7mug] ==> | |
| d | = 12v02/(49mug) |


Next: Statics Up: Completing the Circle Previous: Rolling Motion
larryg@upenn5.hep.upenn.edu
Thu Jan 29 17:25:19 EST 1998 subsubsection4_1_4_4.html